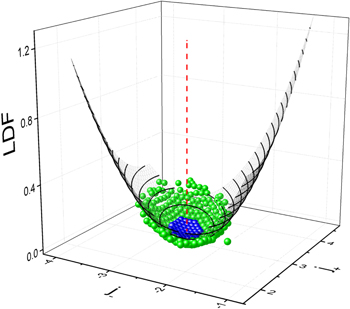
Figure 1. The large deviation functions (LDF) $I({j}_{+},{j}_{-})$ for a two-level Floquet quantum system. The meshed surface is obtained by performing a Legendre transformation on equation (26). The spheres are the data of simulating quantum jump trajectories, where the simulation times for the green and blue data are 50 and 500, respectively. The Fourier transformation of the correlation function is set to be $r(\omega )={ \mathcal A }| \omega {| }^{3}{{ \mathcal N }}_{k}(\omega )$ for ω < 0; otherwise, $r(\omega )={ \mathcal A }| \omega {| }^{3}[{{ \mathcal N }}_{k}(\omega )+1]$, where ${ \mathcal N }(\omega )=1/[\exp (\beta | \omega | )-1]$, and the coefficient A is related to the coupling strength between the system and the heat bath [19]. The parameters used are ω0 = 1, ωR = 0.8, ω = 1.1, ${ \mathcal A }=1$, and β = 1/3. The red dashed line indicates the location of the two mean currents.
|