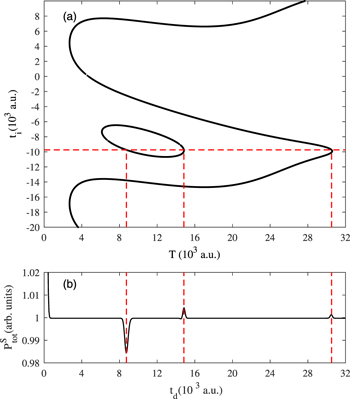
Figure 2. (a) The time period T of the closed orbits from the classical trajectory equation, (5), is plotted against the originating time of the closed orbits ‘ti’ for the fixed parameters Eph = 0.9 eV, β = 0, α = 106.53 a.u., F0 = 100 kV cm−1, ${F}_{1}=3{F}_{0}$ and ${\rm{\Omega }}=2.9489\,\ast {10}^{-4}$ a.u., (b) A plot of the total population based on equation (11) against the time delay td between the two pulses of the double-pulse laser for the peak of the first pulse fixed at tp = −9746 a.u. The total population has peaks at different td. The peaks show the number and structure of the closed orbits originated at the peak of the first pulse. For demonstration, we also show in figure. (a) that the classical periods of the closed orbits originated at the peak of the first pulse i.e. t = tp = −9746 a.u., of the double-pulse laser match the peak position in td.
|