Solving forward and inverse problems of the nonlinear Schrödinger equation with the generalized ${ \mathcal P }{ \mathcal T }$-symmetric Scarf-II potential via PINN deep learning

Jiaheng Li,Biao Li

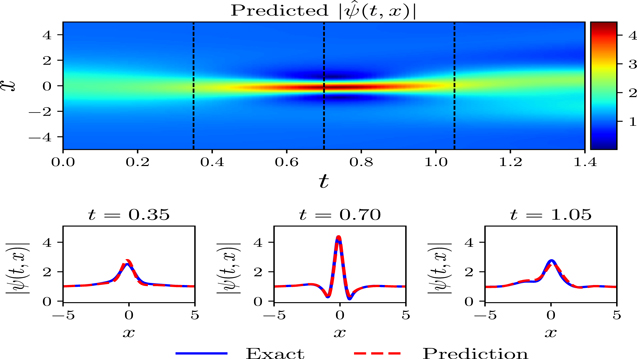
Figure 4. The self-focusing NLSE with the generalized ${ \mathcal P }{ \mathcal T }$-symmetric Scarf-II potential and initial value conditions (16): Top : The magnitude of the predicted solution $| \hat{\psi }(t,x)| $ with ${N}_{{ \mathcal I }}=50$ random sample points with the initial value conditions and ${N}_{{ \mathcal B }}=100$ random sample points with Dirichlet boundary conditions. ${N}_{{ \mathcal C }}=10000$ collocation points are generated by a space-filling LHS strategy. The relative ${{\mathbb{L}}}_{2}$-norm error for this case is 3.110669e-02. Bottom : Comparisons of the exact solutions and predicted solutions at the three temporal snapshots described by the three black dotted lines in the top panel corresponding to time instants t = 2.5, 5.0 and 7.5, respectively.